Periodicity

The following tables indicate the number of symmetry operations of a given period for each point group. If two point groups possess the same elements in the following tables, then they are termed isomorphic. Sets of isomorphic groups are form an abstract group (for example, C_2h, C_2v and D₂).

The period of a symmetry operation is defined as the number of times that operation must be performed to be equivalent to the identity.

Symmetry Operation:	E	Cn	Sn		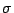i
Period:	1	n	n	2	2

	Period								Period
Point group	1	2	3	4	5	6		Point group	1	2	3	4	5	6
C1	1							C1h	1	1
C2	1	1						C2h	1	3
C3	1		1					C3h	1	1	4
C4	1	1		2				C4h	1	3		4
C5	1				4			C5h	1	1			8
C6	1	1	2			2		C6h	1	3	4			4
	Period								Period
Point group	1	2	3	4	5	6		Point group	1	2	3	4	5	6
S2	1	1						C2v	1	3
								C3v	1	3	2
S4	1	1		2				C4v	1	5		2
								C5v	1	5			2
S6	1	1	2			2		C6v	1	7	2			2
	Period								Period
Point group	1	2	3	4	5	6		Point group	1	2	3	4	5	6
D2	1	3						D2h	1	7
D3	1	2	3					D3h	1	7	4
D4	1	5		2				D4h	1	11		4
D5	1	5			4			D5h	1	11			8
D6	1	7	2			2		D6h	1	15	4			4

	Period								Period
Point group	1	2	3	4	5	6		Point group	1	2	3	4	5	6	8	10	12
T	1	3	8					D2d	1	5		2
Th	1	7	4			4		D3d	1	7	2			2
O	1	9	8	6				D4d	1	9		2			4
Td	1	9	8	6				D5d	1	11			4			4
Oh	1	19	8	12		8		D6d	1	11	2	2		2			4

• Symmetry pages index
• Categorisation by symmetry operations or by unit cell parameters
• Correlation table index
• 3D objects
• Stereographs
• A brief description of tables

Last modified: Tue Dec 4 16:14:35 GMT 2001 by JPG (goss@excc.ex.ac.uk)
V A