Non-crystalographic: D_5h - Products

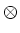Irreducible Representation Products:

product A₁' A₂' E₁' E₂' A₁'' A₂'' E₁'' E₂''

A₁' A₁' A₂' E₁' E₂' A₁'' A₂'' E₁'' E₂''

A₂' A₂' A₁' E₁' E₂' A₂'' A₁'' E₁'' E₂''

E₁' E₁' E₁' A₁'+A₂'+E₂' E₁'+E₂' E₁'' E₁'' A₁''+A₂''+E₂'' E₁''+E₂''

E₂' E₂' E₂' E₁'+E₂' A₁'+A₂'+E₂' E₂'' E₂'' E₁''+E₂'' A₁''+A₂''+E₂''

A₁'' A₁'' A₂'' E₁'' E₂'' A₁' A₂' E₁' E₂'

A₂'' A₂'' A₁'' E₁'' E₂'' A₂' A₁' E₁' E₂'

E₁'' E₁'' E₁'' A₁''+A₂''+E₂'' E₁''+E₂'' E₁' E₁' A₁'+A₂'+E₂' E₁'+E₂'

E₂'' E₂'' E₂'' E₁''+E₂'' A₁''+A₂''+E₂'' E₂' E₂' E₁'+E₂' A₁'+A₂'+E₂'

Dipole Transition Products:

For the D_5h point group, the irreducible representation of the dipole operator is (E₁'+A₂''). Transitions that are dipole forbidden are indicated by parentheses.

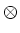product (E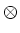₁'+A₂'') product A₁' A₂' E₁' E₂' A₁'' A₂'' E₁'' E₂''

A₁' (E₁'+A₂'') (E₁'+A₁'') A₁'+A₂'+E₂'+E₁'' (E₁'+E₂'+E₂'') (A₂'+E₁'') A₁'+E₁'' (E₁'+A₁''+A₂''+E₂'') (E₂'+E₁''+E₂'')

A₂' (E₁'+A₁'') (E₁'+A₂'') A₁'+A₂'+E₂'+E₁'' (E₁'+E₂'+E₂'') A₁'+E₁'' (A₂'+E₁'') (E₁'+A₁''+A₂''+E₂'') (E₂'+E₁''+E₂'')

E₁' A₁'+A₂'+E₂'+E₁'' A₁'+A₂'+E₂'+E₁'' (3E₁'+E₂'+A₁''+A₂''+E₂'') A₁'+A₂'+E₁'+2E₂'+E₁''+E₂'' (E₁'+A₁''+A₂''+E₂'') (E₁'+A₁''+A₂''+E₂'') A₁'+A₂'+E₂'+3E₁''+E₂'' (E₁'+E₂'+A₁''+A₂''+E₁''+2E₂'')

E₂' (E₁'+E₂'+E₂'') (E₁'+E₂'+E₂'') A₁'+A₂'+E₁'+2E₂'+E₁''+E₂'' (3E₁'+E₂'+A₁''+A₂''+E₂'') (E₂'+E₁''+E₂'') (E₂'+E₁''+E₂'') (E₁'+E₂'+A₁''+A₂''+E₁''+2E₂'') A₁'+A₂'+E₂'+3E₁''+E₂''

A₁'' (A₂'+E₁'') A₁'+E₁'' (E₁'+A₁''+A₂''+E₂'') (E₂'+E₁''+E₂'') (E₁'+A₂'') (E₁'+A₁'') A₁'+A₂'+E₂'+E₁'' (E₁'+E₂'+E₂'')

A₂'' A₁'+E₁'' (A₂'+E₁'') (E₁'+A₁''+A₂''+E₂'') (E₂'+E₁''+E₂'') (E₁'+A₁'') (E₁'+A₂'') A₁'+A₂'+E₂'+E₁'' (E₁'+E₂'+E₂'')

E₁'' (E₁'+A₁''+A₂''+E₂'') (E₁'+A₁''+A₂''+E₂'') A₁'+A₂'+E₂'+3E₁''+E₂'' (E₁'+E₂'+A₁''+A₂''+E₁''+2E₂'') A₁'+A₂'+E₂'+E₁'' A₁'+A₂'+E₂'+E₁'' (3E₁'+E₂'+A₁''+A₂''+E₂'') A₁'+A₂'+E₁'+2E₂'+E₁''+E₂''

E₂'' (E₂'+E₁''+E₂'') (E₂'+E₁''+E₂'') (E₁'+E₂'+A₁''+A₂''+E₁''+2E₂'') A₁'+A₂'+E₂'+3E₁''+E₂'' (E₁'+E₂'+E₂'') (E₁'+E₂'+E₂'') A₁'+A₂'+E₁'+2E₂'+E₁''+E₂'' (3E₁'+E₂'+A₁''+A₂''+E₂'')

Symmetry pages index
Categorisation by symmetry operations or by unit cell parameters
Correlation table index
3D objects
Stereographs
A brief description of tables

	A₁'	A₂'	E₁'	E₂'	A₁''	A₂''	E₁''	E₂''
A₁'	A₁'	A₂'	E₁'	E₂'	A₁''	A₂''	E₁''	E₂''
A₂'	A₂'	A₁'	E₁'	E₂'	A₂''	A₁''	E₁''	E₂''
E₁'	E₁'	E₁'	A₁'+A₂'+E₂'	E₁'+E₂'	E₁''	E₁''	A₁''+A₂''+E₂''	E₁''+E₂''
E₂'	E₂'	E₂'	E₁'+E₂'	A₁'+A₂'+E₂'	E₂''	E₂''	E₁''+E₂''	A₁''+A₂''+E₂''
A₁''	A₁''	A₂''	E₁''	E₂''	A₁'	A₂'	E₁'	E₂'
A₂''	A₂''	A₁''	E₁''	E₂''	A₂'	A₁'	E₁'	E₂'
E₁''	E₁''	E₁''	A₁''+A₂''+E₂''	E₁''+E₂''	E₁'	E₁'	A₁'+A₂'+E₂'	E₁'+E₂'
E₂''	E₂''	E₂''	E₁''+E₂''	A₁''+A₂''+E₂''	E₂'	E₂'	E₁'+E₂'	A₁'+A₂'+E₂'

(E₁'+A₂'')	A₁'	A₂'	E₁'	E₂'	A₁''	A₂''	E₁''	E₂''
A₁'	(E₁'+A₂'')	(E₁'+A₁'')	A₁'+A₂'+E₂'+E₁''	(E₁'+E₂'+E₂'')	(A₂'+E₁'')	A₁'+E₁''	(E₁'+A₁''+A₂''+E₂'')	(E₂'+E₁''+E₂'')
A₂'	(E₁'+A₁'')	(E₁'+A₂'')	A₁'+A₂'+E₂'+E₁''	(E₁'+E₂'+E₂'')	A₁'+E₁''	(A₂'+E₁'')	(E₁'+A₁''+A₂''+E₂'')	(E₂'+E₁''+E₂'')
E₁'	A₁'+A₂'+E₂'+E₁''	A₁'+A₂'+E₂'+E₁''	(3E₁'+E₂'+A₁''+A₂''+E₂'')	A₁'+A₂'+E₁'+2E₂'+E₁''+E₂''	(E₁'+A₁''+A₂''+E₂'')	(E₁'+A₁''+A₂''+E₂'')	A₁'+A₂'+E₂'+3E₁''+E₂''	(E₁'+E₂'+A₁''+A₂''+E₁''+2E₂'')
E₂'	(E₁'+E₂'+E₂'')	(E₁'+E₂'+E₂'')	A₁'+A₂'+E₁'+2E₂'+E₁''+E₂''	(3E₁'+E₂'+A₁''+A₂''+E₂'')	(E₂'+E₁''+E₂'')	(E₂'+E₁''+E₂'')	(E₁'+E₂'+A₁''+A₂''+E₁''+2E₂'')	A₁'+A₂'+E₂'+3E₁''+E₂''
A₁''	(A₂'+E₁'')	A₁'+E₁''	(E₁'+A₁''+A₂''+E₂'')	(E₂'+E₁''+E₂'')	(E₁'+A₂'')	(E₁'+A₁'')	A₁'+A₂'+E₂'+E₁''	(E₁'+E₂'+E₂'')
A₂''	A₁'+E₁''	(A₂'+E₁'')	(E₁'+A₁''+A₂''+E₂'')	(E₂'+E₁''+E₂'')	(E₁'+A₁'')	(E₁'+A₂'')	A₁'+A₂'+E₂'+E₁''	(E₁'+E₂'+E₂'')
E₁''	(E₁'+A₁''+A₂''+E₂'')	(E₁'+A₁''+A₂''+E₂'')	A₁'+A₂'+E₂'+3E₁''+E₂''	(E₁'+E₂'+A₁''+A₂''+E₁''+2E₂'')	A₁'+A₂'+E₂'+E₁''	A₁'+A₂'+E₂'+E₁''	(3E₁'+E₂'+A₁''+A₂''+E₂'')	A₁'+A₂'+E₁'+2E₂'+E₁''+E₂''
E₂''	(E₂'+E₁''+E₂'')	(E₂'+E₁''+E₂'')	(E₁'+E₂'+A₁''+A₂''+E₁''+2E₂'')	A₁'+A₂'+E₂'+3E₁''+E₂''	(E₁'+E₂'+E₂'')	(E₁'+E₂'+E₂'')	A₁'+A₂'+E₁'+2E₂'+E₁''+E₂''	(3E₁'+E₂'+A₁''+A₂''+E₂'')

Non-crystalographic: D5h - Products

Irreducible Representation Products:

Dipole Transition Products:

Last modified: Fri Oct 6 16:30:53 BST 2000 by JPG (goss@excc.ex.ac.uk) V A

Non-crystalographic: D_5h - Products

Last modified: Fri Oct 6 16:30:53 BST 2000 by JPG (goss@excc.ex.ac.uk)
V A